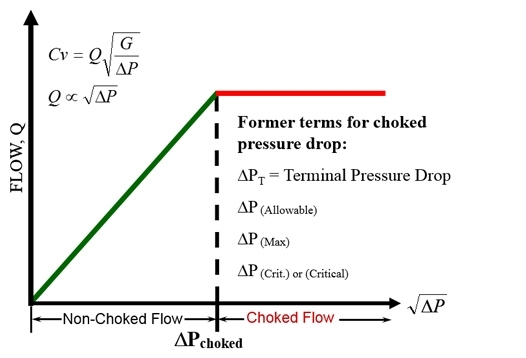
Choked FlowThe basic liquid sizing equation, shown in the upper left of Figure 1, tells us that the liquid flow rate through a control valve is proportional to the square root of pressure drop. This simple relationship is shown graphically by the green portion of the graph in Figure 1. (Note that the scale of the horizontal axis is the square root of pressure drop.) This linear relationship does not always hold true. As the pressure drop is increased by lowering the downstream pressure, the flow reaches a point where it no longer increases. Once this happens, additional increases in pressure drop across the valve do not result in additional flow, and flow is said to be choked. Here we will call this limiting or choking pressure drop ΔP
choked, so as to be in agreement with the latest versions of the IEC and ISA control valve sizing equation standards. (Both standards are technically identical.) Prior to the issuance of the 2011 edition of the IEC valve sizing equation standard and the 2012 version of the ISA valve sizing equation standard there was no official name given to the dividing line between non-choked flow and choked flow, so valve manufacturers made up their own names. Some of the most common ones are listed in Figure 1.
Figure 1. Liquid flow in a control valve as a function of the pressure drop across the valve.
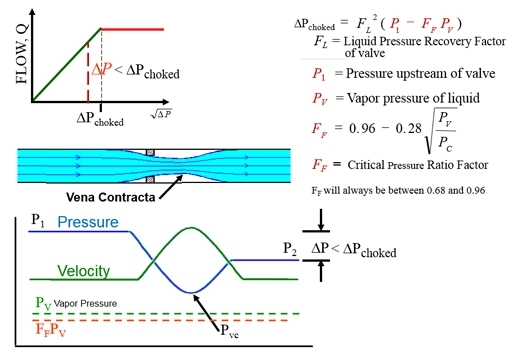
Let’s take a look at what is happening inside the valve to cause this choking of the flow. When the flow stream passes through the vena contracta (the point at which the cross sectional area of the stream is at a minimum), the flow velocity reaches a maximum. Conservation of energy dictates that since kinetic energy at the vena contracta has increased to a maximum, potential energy, in the form of static pressure, must decrease to a minimum. This is illustrated graphically in the lower left of Figure 2. Note that in the figure, ΔP is less than ΔP
choked and flow is not choked.
Figure 2. Velocity and pressure profile in a control valve without choked flow.
With a liquid at rest, lowering the surrounding pressure to the vapor pressure of the liquid will cause the liquid to begin vaporizing. Because any particular group of liquid molecules is in the vena contracta for a very short time, experimentation has shown that the pressure at the vena contracta must actually drop slightly below the vapor pressure of the liquid before vaporization begins. The amount below the vapor pressure to which the vena contracta pressure must drop for vaporization to begin and flow to choke is approximated in the IEC and ISA Standards by the
Liquid Critical Pressure Ratio Factor, F
F. Figure 2 shows the ISA and IEC equation that is used to calculate F
F. So, in the ISA/IEC equation that calculates the value of ΔP
choked, the vapor pressure of the liquid is multiplied by F
F or as it is written in the equation for ΔP
choked, “F
F P
V.” It should be noted that very often in text books and manufacturer’s literature the subject of the Liquid Critical Pressure Ratio Factor is not mentioned, but it is this writer’s opinion that the very slight increase in complexity here is justified in order to give the reader a fuller understanding of the subject of liquid flow in control valves.
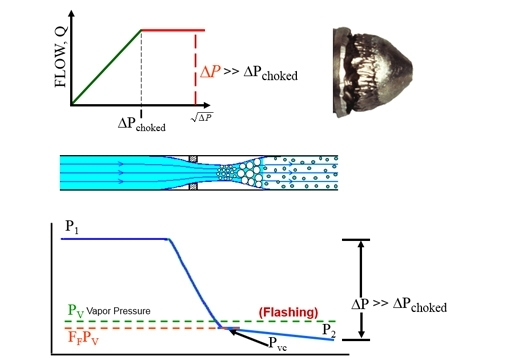
If flow increases to the point that the vena contracta pressure drops to F
F P
V, vapor bubbles form in the vena contracta. Any additional decrease in the downstream pressure causes more bubbles to form, but the pressure at the vena contracta does not decrease below F
F P
V. At this point it is worth noting that the flow through a control valve depends on the pressure difference between P
1 and P
vc (the pressure at the vena contracta), and since the vena contracta pressure does not decrease below F
F P
V, the flow does not increase, resulting in choked flow. Figure 3 illustrates the choking process along with the cavitation discussed in the next paragraph. Note that in the figure, ΔP is greater than ΔP
choked; and flow is choked.
CavitationAs the bubbles move down stream, the cross-sectional flow area opens up, the velocity goes down and the pressure goes up. Now we have bubbles with an internal pressure equal to the vapor pressure surrounded by a higher pressure. The bubbles collapse in on themselves. This combination of bubble formation and the resulting choked flow, along with the collapse of the bubbles downstream is called CAVITATION. When the bubbles collapse they make a popping sound. The result is a noise like gravel going through the valve. This noise can be loud enough to be very annoying and even loud enough to damage the hearing of a person who is exposed to it for long periods. Also, when the bubbles collapse, they create shock waves that can cause severe damage to the valve. The appearance of cavitation damage is a rough, cinder like, look. (See the picture of a globe valve plug in the upper right side of Figure 3.) This damage can happen very quickly, sometimes in as little as a few weeks or months. Because cavitation damage happens so quickly, we try to avoid cavitation at all costs. Very hard materials give some improvement, but usually the improved performance is not enough to justify the cost.
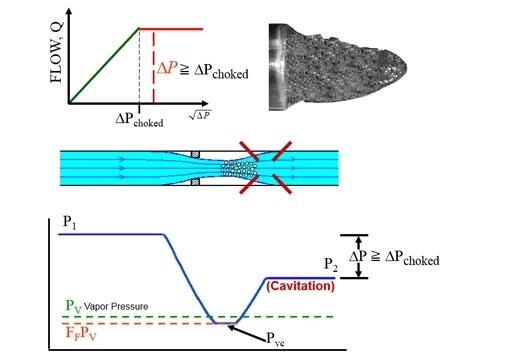
Figure 3. Velocity and pressure profile in a control valve with choked flow and cavitation.
FlashingIf we continue to decrease the downstream pressure, we reach a point where the pressure downstream of the valve is less than the vapor pressure of the liquid and we have the situation shown in Figure 4.
Figure 4. Velocity and pressure profile in a control valve with choked flow and flashing.
Now, instead of collapsing, the bubbles become larger and very soon transition from liquid with bubbles in it to vapor with small drops of liquid in it. This is called FLASHING. The appearance of flashing damage is quite different from cavitation damage, and appears as smooth, shiny rivers and valleys. (See the picture of a globe valve plug in the upper right side of Figure 4.) The damage mechanism is a sand blasting effect. Downstream of the vena contracta the flow consists of a large volume of vapor with many tiny drops of liquid. Because the volume increases greatly when liquid vaporizes, the downstream velocity can be several hundred feet per second, and the high velocity liquid droplets can erode away a valve part. The damage caused by flashing does not usually happen as quickly as that caused by cavitation. The use of hard or erosion resistant materials can often bring the damage to within tolerable limits. Trim parts made of the hard stainless steels, such as 17-4 ph, hold up quite well, and 316ss or chrome moly bodies do much better than carbon steel. The existence of flashing conditions is dictated by the system (P
2 is less than P
v) and the valve selection neither causes or prevents flashing. The noise caused by flashing is usually below 85 dBA and to the author’s knowledge there is no method for calculating flashing noise.
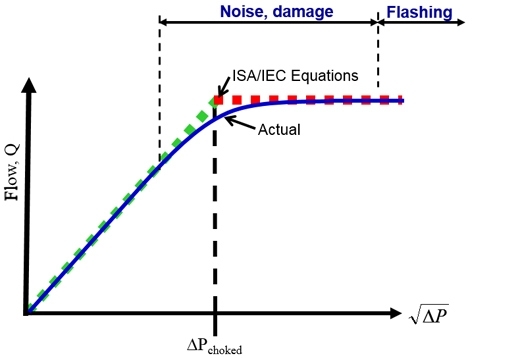
The Real SituationFigure 1 and the associated discussion of liquid choked flow is the classical discussion, and implies that there is a sudden transition from non-choked flow to fully choked flow. In reality, at pressure drops approaching, but below the calculated value of ΔP
choked, there is usually some formation of vapor bubbles and some degree of cavitation. Figure 5 shows what really happens as flow transitions from non-choked to fully choked flow. Figure 5 shows what really happens as flow transitions from non-choked to fully choked flow.
Figure 5. Actual transition between non-choked and choked flow.
The length of the transition is a function of the shape of the primary restriction in the valve. Many rotary vales have irregular cross-sectional flow areas which can result in substantial amounts of choking and cavitation at lower operating pressure drops which can begin in one localized region and gradually spread to the entire restriction as the pressure drop across the valve increases and flow becomes fully choked. The restriction in most globe valves is quite symmetrical, resulting in a shorter transition. The current ISA and IEC control valve sizing methods do not include a method of calculating where the transition from non-choked to fully choked flow begins and ends and only give formulas for calculating the red and green lines in Figures 1 and 5. Figure 5 also indicates that noise and cavitation damage can begin even before the flow curve begins to deviate from a straight line. These first stages of cavitation begin when the average pressure in the main flow jet at the vena contracta is still above F
F P
V. At points of abrupt increase in flow area the streamlines that are attached to the physical boundaries of the valve can separate and when they do, they form vortices or eddies. The rotational velocity in the eddies can be high enough that the local pressure inside an eddy drops below the vapor pressure and vapor bubbles form. As the eddy’s rotational velocity decreases, the pressure surrounding the vapor bubbles increases and the bubbles collapse causing both noise and damage.
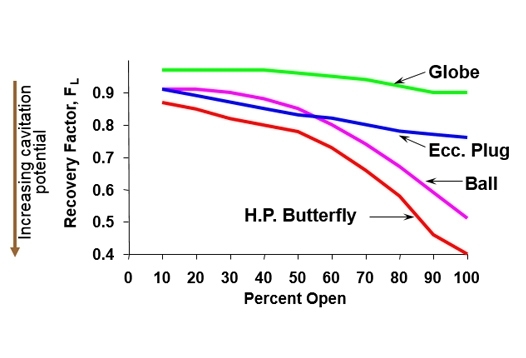
The value of ΔP
choked is a function of both the process conditions (P
1, the pressure upstream of the valve and P
v, the vapor pressure of the liquid) and the valve’s internal geometry represented by the experimentally determined Liquid Pressure Recovery Factor, F
L. Typical values of F
L are shown in Figure 6. Note that F
L is a function of both valve style and the percentage of valve opening. Higher values of F
L are associated with valves that have a lower potential for choked flow and cavitation, and smaller values of F
L are associated with valves that have a greater potential for choked flow and cavitation.
There are several methods of increasing the value of ΔP
choked and thus reducing the potential for cavitation and the associated noise and damage: (1) The value of P
1 can be increased while keeping ΔP the same by moving the control valve to a location further upstream, or to a location at a lower elevation. (2) The vapor pressure can be decreased by installing the valve where the liquid temperature is lower, such as the cool side of a heat exchanger. (3) A valve style with a higher value of F
L can be selected. It is interesting to note that in general, as the F
L increases, so does the price of the valve. There are special cavitation resistant adaptations of many of the valve styles that have larger values of F
L than those shown in Figure 6, yet which retain the other desirable features of that style.
Figure 6. Typical values of the Liquid Pressure Recovery factor, FL.
Before actually purchasing a control valve, it is always a good idea to ask for the manufacturer’s or the manufacturer’s representative’s comments regarding your selection.
A more extensive discussion of liquid flow in control valves can be found in Chapter 4 of Valin Corporation’s book, Control Valve Application Technology.
Here are links to white papers that may be of interest:Pressure at the Vena Contracta with Liquid Flow in a Control ValveInstalled Gain as a Control Valve Sizing CriterionAerodynamic Noise in Control ValvesValve Aerodynamic Noise Reduction StrategiesDetermining the Pressure Drop to be Used in a Control Valve Sizing CalculationSize Matters: Control Valve Sizing 101The content of these white papers are just a small portion of what you will learn in Dr. Monsen's book:
Control Valve Application TechnologyWould you rather learn from Dr. Monsen directly and have the ability to ask him questions? Take one or more of his webinars: