Resources
Installed Gain as a Control Valve Sizing Criterion
Submitted by Jon Monsen, Ph.D.
For most systems, in order to get good control with stability throughout the full range of required flow rates, we need to use a control valve that has an installed flow characteristic that is linear, or at least as close to linear as possible. It is often difficult to compare the control capability of two valves with less than perfectly linear installed characteristics by simply studying their installed characteristic graphs, and you can learn more about how well they will control a particular system by examining their installed gain.
Installed Gain
The left hand graph in Figures1, 2 and 3 is an installed characteristic, and the right hand graph is the corresponding installed gain.

Figure 1. The installed gain of a control valve with an ideal perfectly linear installed characteristic.
The gain of a device is defined as the ratio of the change in output to the corresponding change in input.
In the case of a control valve, the output is the flow in the system (q) and the input is valve position (h).
A graphical interpretation of the GAIN is the SLOPE of the installed characteristic.
In the eighth grade we learned that the slope of a line is defined as the RISE divided by the RUN. In the case of a control valve, the RISE is the change in flow and the RUN is the change in valve stem position. For an ideal linear installed characteristic, for any given change in valve travel, the flow will change by the same amount, Δq/Δh = Gain = 1.0 at all points. (See Figure 1.)
So for the ideal linear installed characteristic the ideal installed gain graph is a constant 1.0.
We will never exactly get the ideal installed characteristic and installed gain: (1) because real valves do not have exactly linear or equal percentage inherent characteristics, and (2) because the interaction between the equal percentage inherent characteristic and the system characteristic do not exactly cancel each other, but we want to get as close as we can, so the perfectly linear installed characteristic and the constant installed gain of 1.0 are the benchmark we always aim for.
In Figure 2 we have two valves with straight line installed characteristics, one with a very steep slope and one with a very shallow slope.
The valve with the green graph, whose installed characteristic has a steep slope is a very sensitive valve. Its gain graphs as a constant, but large, number.
The valve with the red graph, whose installed characteristic has a shallow slope, is a very insensitive valve. Its gain graphs as a constant, but small, number.
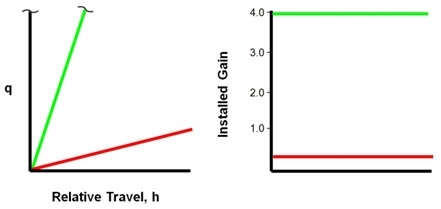
Figure 2. Two valves with straight line installed characteristics. One with a steep slope and a high gain, and one with a shallow slope and a low gain.
It turns out that neither of these valves would make a very good control valve.
The low gain valve would not make a good control valve, because when the valve stem moves, the flow hardly changes at all. A control valve, that when it moves does not change the flow, is not much of a control valve!
The valve with the steep slope has a very high gain, meaning that small changes in valve position cause very large changes in flow. It is less obvious why this valve would not be a good control valve. When two parts (such as a ball and a seat, or a valve shaft and packing) are in contact with each other, they exhibit two kinds of friction. When the parts are not moving, they tend to stick together and the friction is high. When they are moving the friction becomes much lower. The interaction between static and dynamic friction makes it very difficult to position a valve exactly where you want it.
It is fairly common to find installed valves that you cannot position much more accurately than within one percent of where you want them. From the definition of gain, the change in flow will be equal to the change in position multiplied by the installed gain (Δq = Δh * Gain). If the high gain valve (installed gain of 4) could only be positioned in one percent increments, the most accurately that flow could be controlled would be in 4 percent increments, which might not give accurate enough control.
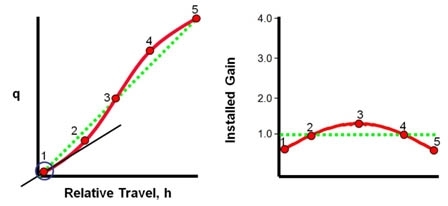
Figure 3. Installed characteristic and installed gain of an equal percentage valve installed in a system with a lot of pipe.
Now that we have discussed the meaning of installed gain, we can extend our discussion to the real world of an equal percentage valve installed in a system with a lot of pipe, where the installed characteristic is nearly linear, but slightly “S” shaped as shown in Figure 3.
We have left dashed lines to represent the ideal linear installed characteristic and the resulting ideal installed gain with a constant value of 1.0.
Here the shape of the installed characteristic graph is constantly changing and its slope is also constantly changing.
Let’s take a look at the instantaneous slope (and thus the gain) at several points.
At Point 1, a line has been drawn that is tangent to the installed characteristic to represent the instantaneous slope of the installed characteristic (and thus the installed gain) at Point 1.
This tangent is not as steep as the ideal linear installed characteristic, and therefore the gain is less than the ideal 1.0. A point has been placed on the Installed gain graph (Point 1) that is less than the ideal gain of 1.0.
At Point 2, if we were to draw a tangent to the installed characteristic graph, it would be parallel to the ideal linear graph, so at Point 2 the instantaneous gain is 1.0 and a corresponding Point 2 is placed on the installed gain graph at a gain of 1.0.
At Point 3, , if we were to draw a tangent to the installed characteristic graph, it would have a steeper slope than the ideal linear graph, so at Point 3 the instantaneous gain is greater than 1.0, and a corresponding Point 3 is placed on the Installed gain graph.
At Point 4, if we were to draw a tangent to the installed characteristic graph, it would be parallel to the ideal linear graph, so at Point 4 the instantaneous gain is 1.0 and a corresponding Point 4 is placed on the installed gain graph at a gain of 1.0.
At Point 5, if we were to draw a tangent to the installed characteristic graph, it would not be as steep as the ideal linear characteristic, so at Point 5, the instantaneous gain is less than 1.0 and a corresponding Point 5 is placed on the installed gain graph.
Typically, the installed characteristic and installed gain graphs of equal percentage valves in systems with a lot of pipe (the most common case) will have shapes similar to those in Figure 3, but sometimes not as symmetrical as shown here.
Installed Gain Recommendations
Below are our recommendations (and the rules that Nelprof, the Neles control valve sizing and selection software, uses when selecting the best valve size for an application) for gain magnitude and variation.
Within the specified control range:
1. Gain > 0.5
2. Gain < 3.0
3. Gain (max) / Gain (min) < 2.0
4. As constant as possible
5. As close to 1.0 as possible
2. Gain < 3.0
3. Gain (max) / Gain (min) < 2.0
4. As constant as possible
5. As close to 1.0 as possible
Within the specified control range (by definition we will not be controlling outside this range so we are not concerned with what happens there), that is between qmin and qmax, the gain should not be less than 0.5, or greater than 3.0. (See the left hand graph in Figure 7-4 for a graphical representation of Criteria 1, 2 and 3.)
Going back to the definition of gain, that is the change in flow equals the change in valve position multiplied by the gain (Δq = Δh * Gain), if the gain is too low, when the valve moves the flow hardly changes, which means the valve will not be effective in controlling flow. If the gain is too high, small errors in valve position will result in large errors in flow, making it difficult or impossible to control accurately.

Figure 4. Left, gain criteria 1, 2, and 3 shown graphically. Right, system response when gain change is 2.0 or less.
Typically, if the gain changes by not much more than a 2 to 1 ratio, it will be possible to come up with one set of PID tuning parameters that will result in good control and stability throughout the required flow range.
The right hand graph in Figure 4 shows the system response at the point of minimum gain and at the point of maximum gain in a system where the gain change within the specified flow range is 2 to 1.
In Figure 5, the gain within the specified control range is changing by almost a six to one ratio.
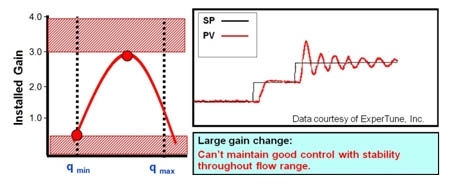
Figure 5. System response when the gain change is approximately 6 to 1.
If the PID controller is tuned when the system is operating at the point of minimum gain, then the set point is stepped we get a quick stable response (as we should, since this is the point where the loop was tuned).
Later, when the system is operating at the point of maximum gain, if we step the set point we get an oscillatory response because the PID parameters that were suitable for a low gain system are way too aggressive when the gain is much higher.
With large gain variation, if the loop is tuned where the gain is low, the loop becomes unstable where the gain is higher. If it is tuned for stability at the point where the gain is highest, control will be slow and sluggish when operating in the lower gain regions.
If you can’t find any valve that meets the first three criteria, or if you want to select the best valve of several that all meet the first three, then use criteria 4 and 5.
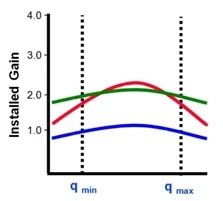
Figure 6. Applying Criteria 4 and 5.
The gain should be as constant as possible. The more constant the gain, the more aggressive can be the PID tuning without the danger of instability. Refer to Figure 6. If you had the choice between the green valve and the red valve, the green valve would be the best choice because the PID tuning could be more aggressive.
The gain should also be as close to 1 as possible. The green valve and the blue valve both allow equally aggressive tuning, but the blue valve is a better choice.
For a valve position error of 1%, the green valve would give a flow error of about 2% and the blue valve would give a flow error of about 1%.
Usually, when comparing the installed gain of different valves for the same application, as the gain becomes more constant it also comes closer to 1.
Program Graphs Installed Characteristic and Gain
Next, we will look at a control valve sizing program that, based on a database of actual valve inherent characteristics, along with some user supplied information about how the system pressure drop changes with flow, can calculate and graph the installed flow characteristic of a particular type and size valve in the system it will be used in. Then the program calculates the first derivative of the installed flow characteristic and graphs it as the installed gain.
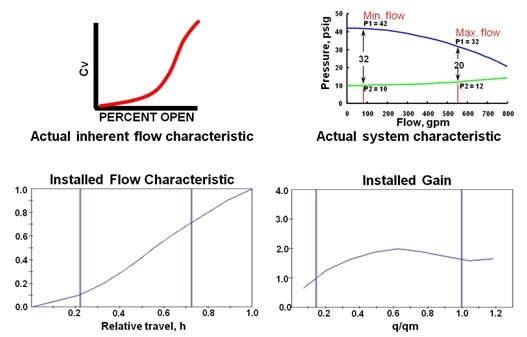
Figure 7. Program graphs the installed characteristic and gain based on tables of inherent flow characteristics, and user supplied information on the system characteristic.
In order for the program to define the process model, at least two flow points (maximum and minimum flow), along with the associated values of pressure upstream of the control valve, P1, and the pressure drop across the control valve, ΔP, are required.
Using Nelprof, the Neles control valve sizing and selection software, we will demonstrate how an analysis of installed gain can help select the best control valve for a particular system. The demonstration is based on the system shown in Figure 8.
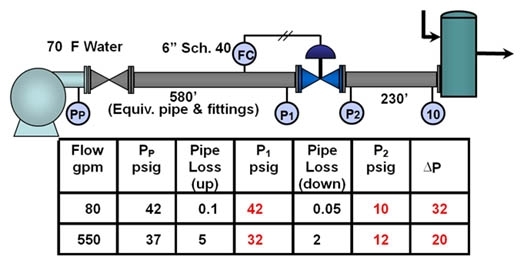
Figure 8. Flow control loop used to demonstrate how an analysis of control valve installed gain can help select the valve that will give the best control.
The task is to select a properly sized SEGMENT BALL control valve (which has an equal percentage inherent flow characteristic). Besides minimum and maximum flow rates, the computer program needs to know the pressure drop across the control valve at each of these flow rates.
The data relating to control valve pressure drop shown in Figure 8 was determined as follows: Start at a point upstream of the valve where the pressure is known, then at the given flow rate, subtract the system pressure losses until you reach the valve inlet, at which point you have determined P1. Then go downstream until you find another point where you know the pressure, then at the given flow rate work backward (upstream) adding (you add because you are moving upstream against the flow) the system pressure losses until you reach the valve outlet at which point you have determined P2. You can now subtract P2 from P1 to obtain ΔP.
The graph in the upper right of Figure 7 shows how P1, P2 and ΔP vary with flow as modeled by the software. The shapes of the P1 and P2 curves are determined by the software by obtaining a least squares curve fit to the given values of P1 and P2, knowing that the curves will be parabolic and will have a slope of zero at zero flow.
Figure 9 shows the installed flow characteristic and installed gain graphs for three possible sizes of segment ball valve.
As the valve size gets smaller the characteristic will become more linear and the gain will get lower and more constant. (The goal is to select a valve whose installed gain is as constant as possible, and as close to 1.0 as possible.)
For this example, things like choked flow, noise, and velocity do not affect the selection, allowing us to concentrate on installed characteristics and gain. The graphs below are what you would see if you performed the sizing calculations for a 6 inch, a 4 inch and a 3 inch Neles RE Series segment ball valve.
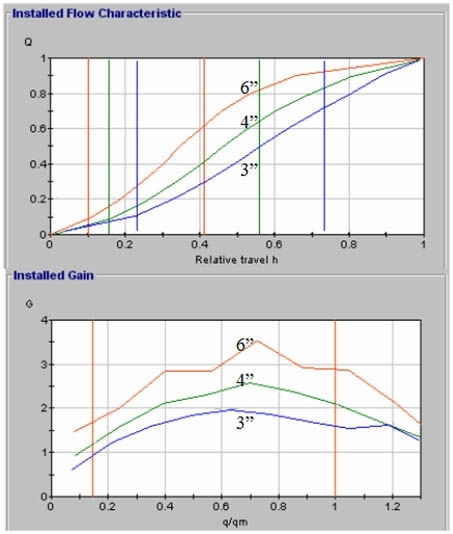
Figure 9. Installed flow characteristic (top) and installed gain (bottom) for three possible sizes of segment ball valves in the system of Figure 8.
The top graph is the installed characteristics of the three sizes and the top trace (red) represents the 6 inch RE Series segment ball valve installed in the system shown in Figure 8. The Nelprof program inserts two vertical lines to show the location of the specified minimum and maximum flows (in this example, 80 and 550 gpm). The red (first and fourth) vertical lines go with the 6 inch valve. You can see that on the low end, there is not a lot of safety factor. You can also see that we are only using about 42% of the valve's capacity. The rest of the valve's capacity is not being used, meaning that a 6 inch valve is larger than required for the application. The effect of decreasing pressure drop across the control valve with increasing flow has resulted in this equal percentage valve having a fairly linear installed characteristic, especially within the specified flow range of 80 to 550 gpm.
The bottom graph is the valve's installed gain (a graph of the instantaneous slope of the characteristic graph). The gain graph gives us a better idea of how well the valve will be able to control.
Looking at the installed gain graph for the 6 inch valve, we see that the gain varies by slightly more than 2:1 within the specified control range, slightly more than the criteria we recommend. The more the gain changes within the control range the more difficult it is to tune the control loop for both quick response and stable operation. Also, the gain has a maximum value of 3.5 which is higher than recommended by Criterion 2. Note that the horizontal scale on the gain graph has units of q/qm (actual flow divided by the maximum specified flow). This means that at "1" on the scale, flow is 100% of the maximum specified flow of 550 gpm. (This is why there is only one set of vertical lines on the gain graph, because the horizontal scale on the gain graph is always 0 to 130% of the maximum specified flow - in this example 550 gpm.)
The maximum gain of 3.5 occurs at q/qm of 0.7 or 70% of 550 gpm. At this point, a position error of 1% would cause a flow error of 3.5%. This would make it difficult for the system to control accurately.
The conclusion from examining the installed characteristic and gain graphs is that the 6 inch segment ball valve is not an ideal choice for this system.
The graphs in green represent the installed characteristic and gain for a 4 inch segment ball valve. Because the horizontal axis for the installed characteristic graph is valve travel, the vertical green (second and fifth) lines representing 80 and 550 gpm for the 4 inch valve are both at larger openings for the smaller valve. The installed characteristic is quite linear within the specified flow range, which is good. The 4 inch valve has more safety factor on the low end, and less wasted capacity on the high end. You can get an even better idea of the improvement of the 4 inch valve over the 6 inch valve by looking at the installed gain graph. The maximum gain of the 4 inch valve is lower, meaning for the same position error, the flow error will be less. Also the change in gain from its lowest value within the specified flow range and its highest value is slightly less, meaning that it would be possible to use slightly more aggressive tuning for the PID controller, resulting in better control.
The conclusion is that the 4 inch valve is a better choice than the 6 inch valve.
The graphs in blue represent the installed characteristic and gain of a 3 inch segment ball valve. The minimum and maximum specified flows of 80 and 550 gpm, represented by the vertical blue (third and sixth) lines on the installed characteristic graph, are symmetrically located on the installed characteristic, resulting in nearly equal safety factors on both the low end and the high end. The installed characteristic is also quite linear within the specified flow range. The installed gain graph has the lowest maximum value of all three valves, which will result in the lowest flow error for the same position error. The variation in gain within the specified flow range is the least of all three valves, making possible the most aggressive PID tuning.
The conclusion is that of the three valves analyzed, the 3 inch valve will provide the best control.
Pressure at the Vena Contracta with Liquid Flow in a Control Valve
Aerodynamic Noise in Control Valves
Valve Aerodynamic Noise Reduction Strategies
Determining the Pressure Drop to be Used in a Control Valve Sizing Calculation
Size Matters: Control Valve Sizing 101
The content of these white papers are just a small portion of what you will learn in Dr. Monsen's book: Control Valve Application Technology
Would you rather learn from Dr. Monsen directly and have the ability to ask him questions? Take one or more of his webinars:
- Control Valve Overview
- Effect of Control Valve Sizing and Flow Characteristic on Controllability
- Liquid Flow in Control Valves
- Gas Flow and Aerodynamic Noise in Control Valves
- Control Valves and Process Variability
- Installed Gain as a Control Valve Selection Criterion
A lesson for me is that I need to involve you earlier in the program.
You were tireless in your support and it will not be forgotten!

