Resources
Calculating Rotary and Electrical Power
Submitted by Parker Electromechanical
The scientific law of conservation of energy dictates that energy cannot be created nor destroyed, but it can be converted. In other words energy that is used in some given situation must come from another source of energy. It cannot come from “thin air.” However, energy comes in many forms and can be exchanged from one form to another (i.e. chemical, thermal, gravitational, among many others).
Likewise, mechanical rotary power given from a motor must be driven by an appropriate amount of electrical power provided by the drive. Like the energy example, rotary power is provided for by the electrical input power, and, also like the energy example, the rotary power cannot exceed the electrical. Therefore, it is important to verify the power throughout the system from power supply all the way to the motor or gearbox output shaft.
Note that this article assumes that the reader has a foundational understanding of Ohm’s Law (V=IR), conservation of energy, and algebraic operations.
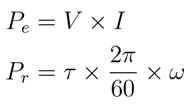 Where Pe is electrical power and Pr is rotary power, V is voltage, I is current, τ is torque in Nm, and ω is speed in RPM.
Where Pe is electrical power and Pr is rotary power, V is voltage, I is current, τ is torque in Nm, and ω is speed in RPM.
Current supplied to the drive is DC current, but the current is processed into a sinusoidal wave to drive the motor. Unfortunately, there are different methods to process that signal, and therefore, this step requires investigating the type of current rating the drive uses. Find the continuous current limit for the drive and whether it is peak-of-sine or RMS. The power equation is in terms of peak-of-sine so if the current rating is in RMS then you will need to multiply by the square root of 2.
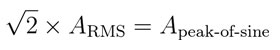 Multiply VDC by the rated current in terms of peak-of-sine to find the electrical power that the drive is capable of.
Multiply VDC by the rated current in terms of peak-of-sine to find the electrical power that the drive is capable of.
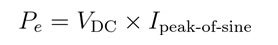
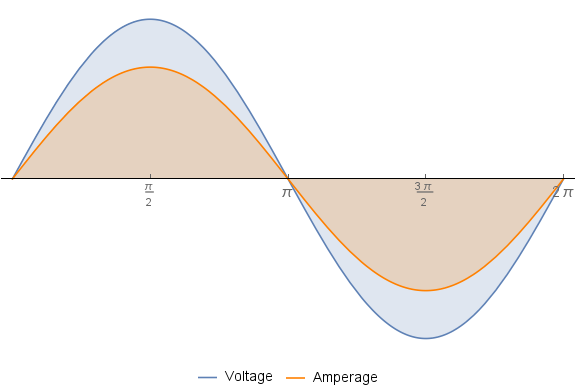
 And this equation is related to the graphic below amperage oscillations are in sync with voltage oscillations.
And this equation is related to the graphic below amperage oscillations are in sync with voltage oscillations.
Voltage and current are both defined as RMS, but what if your drive is specified in terms of peak-of-sine? Divide the peak-of-sine value by the square root of 2 to find the equivalent RMS value as shown in the previous section.

To give some background on transformers, they come in many shapes and sizes but basically use coiled copper to change or ‘transform’ the power signal. Unfortunately, there is a phase shift introduced due to the nature of current flowing through coiled wire and the property of inductance.
When the voltage and amperage are out of phase the available power is reduced by the cosine of the phase shift angle. Therefore, the power equation is extended to the following form:
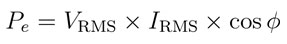 Note that this only applies if there is a phase shift between voltage and current! If the phases of the voltage and current are aligned (i.e. zero phase shift) then the cosine term goes to 1.
Note that this only applies if there is a phase shift between voltage and current! If the phases of the voltage and current are aligned (i.e. zero phase shift) then the cosine term goes to 1.
The following plot graphically describes what a phase shift would look like.
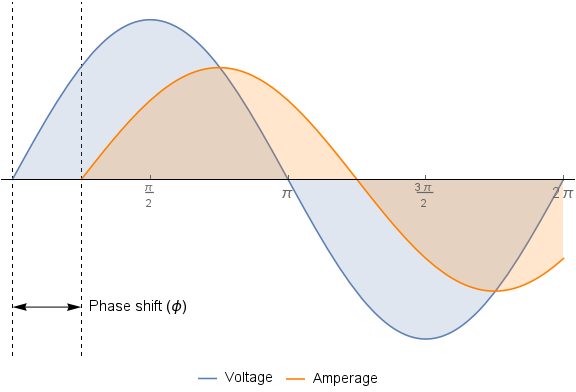
Unfortunately, the effect that a phase shift has on a system can only be quantified empirically through experimentation. Prediction of the phase shift is well beyond the level of this article, and as such this topic is mentioned only make the reader aware of its existence and influence on the system.
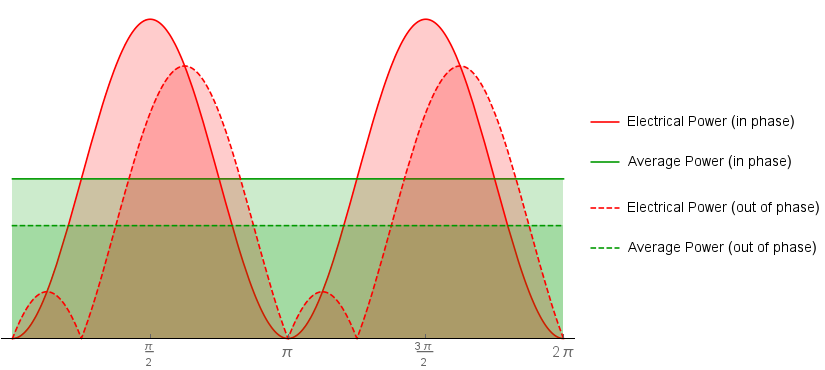
The plot below compares the instantaneous and average power between the in-phase and out-of-phase condition. One can rather easily see that the phase-shifted average power is less.
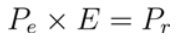 The general equation above accounts for losses in the drive including phase shift, resistive losses in the wiring, and electrical and mechanical losses in the motor. Therefore, the E is not just one number; the total system efficiency is a product of the efficiencies of each component.
The general equation above accounts for losses in the drive including phase shift, resistive losses in the wiring, and electrical and mechanical losses in the motor. Therefore, the E is not just one number; the total system efficiency is a product of the efficiencies of each component.
 Typical efficiency of each component:
Typical efficiency of each component:
Therefore, the total available rotary output power is equal to the electrical input power times the total efficiency. Keep in mind that this available output power is a best-case scenario since other small losses have been neglected.
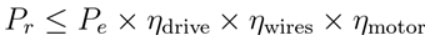
Motors have an extensive efficiency range that depends on multiple variables including winding specifications, running speed and torque, and ambient temperature among others. The motor’s performance documentation will sometimes include this.
Phase shift losses will be determined entirely by the electrical components used in the drive’s construction. The amount of phase shift in any given drive can only be quantified empirically through experimentation. Further details of phase shift effects are well beyond the scope of this article.
Gearheads are commonly attached to motors for many reasons, namely to gain a mechanical advantage or reduce cogging effects. Thankfully, the same power equation that is applicable to motors also applies to gearheads; one just needs to account for the efficiency of the gearhead in addition to all the other components. The power balance equation is extended to the following equation where Pr refers to the gearhead output rather than the motor output:
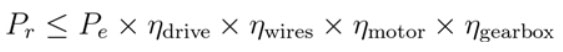 CALCULATING CURRENT DRAWN FROM POWER SUPPLY TO DRIVE
CALCULATING CURRENT DRAWN FROM POWER SUPPLY TO DRIVE
Understanding the previous equations allows a rough calculation of the current drawn from the power supply. In the case of a DC power supply:
 And in the case of an AC power supply:
And in the case of an AC power supply:
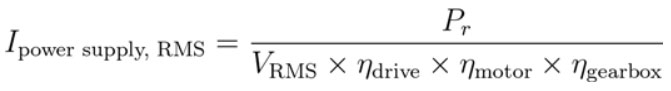
Likewise, mechanical rotary power given from a motor must be driven by an appropriate amount of electrical power provided by the drive. Like the energy example, rotary power is provided for by the electrical input power, and, also like the energy example, the rotary power cannot exceed the electrical. Therefore, it is important to verify the power throughout the system from power supply all the way to the motor or gearbox output shaft.
Note that this article assumes that the reader has a foundational understanding of Ohm’s Law (V=IR), conservation of energy, and algebraic operations.
FOUNDATIONS
The foundational power equations are as follows:ROTARY POWER
Mechanical power is a force exerted through a distance over some period of time. The rotational version is torque exerted through some angle over some time period. Therefore, multiplying torque by speed and an additional conversion factor results in mechanical rotary power, Pr.ELECTRICAL POWER
Electrical power, Pe, is voltage multiplied by current; however, due to the variable nature of electricity it is commonly not this simple. There are three forms of electrical power that your application could be classified into.1.) DC POWER SUPPLY
This is the most straightforward form of electricity and calculation is relatively simple. The voltage term in the power equation is simply the DC voltage input value.Current supplied to the drive is DC current, but the current is processed into a sinusoidal wave to drive the motor. Unfortunately, there are different methods to process that signal, and therefore, this step requires investigating the type of current rating the drive uses. Find the continuous current limit for the drive and whether it is peak-of-sine or RMS. The power equation is in terms of peak-of-sine so if the current rating is in RMS then you will need to multiply by the square root of 2.
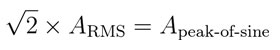
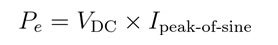
2.A.) AC POWER SUPPLY (IN PHASE)
The governing electrical power equation for AC is more complex because of the ‘alternating’ nature of the current. The most simplistic power formula for alternating current is as follows:

Voltage and current are both defined as RMS, but what if your drive is specified in terms of peak-of-sine? Divide the peak-of-sine value by the square root of 2 to find the equivalent RMS value as shown in the previous section.
2.B.) AC POWER SUPPLY (PHASE SHIFTED)
Sometimes, however, voltage and current are not synchronized as shown in the previous section. One situation this can occur is when a standard transformer is used to rectify the alternating current brought into a drive. Below is an example of a traditional transformer.
To give some background on transformers, they come in many shapes and sizes but basically use coiled copper to change or ‘transform’ the power signal. Unfortunately, there is a phase shift introduced due to the nature of current flowing through coiled wire and the property of inductance.
When the voltage and amperage are out of phase the available power is reduced by the cosine of the phase shift angle. Therefore, the power equation is extended to the following form:
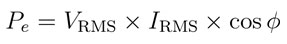
The following plot graphically describes what a phase shift would look like.

Unfortunately, the effect that a phase shift has on a system can only be quantified empirically through experimentation. Prediction of the phase shift is well beyond the level of this article, and as such this topic is mentioned only make the reader aware of its existence and influence on the system.
The plot below compares the instantaneous and average power between the in-phase and out-of-phase condition. One can rather easily see that the phase-shifted average power is less.

POWER BALANCE
We can now look at the efficiency of a system. Recall that the laws of nature require that power out cannot be greater than power in. Since they also dictate that a system cannot be perfectly efficient, there must be some term introduced that accounts for this efficiency loss.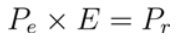

- ηdrive: about 95%
- ηwires: typically neglected
- ηmotor: widely variable from 50% to 90% and dependent on the motor's speed, torque, and winding parameters
Therefore, the total available rotary output power is equal to the electrical input power times the total efficiency. Keep in mind that this available output power is a best-case scenario since other small losses have been neglected.
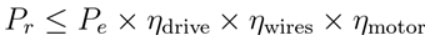
WHERE TO FIND THE EFFICIENCY DATA
Drive and gearbox efficiencies are often published in catalogs and datasheets. Drives are in the realm of 95% while most all planetary gearboxes are above 90%.Motors have an extensive efficiency range that depends on multiple variables including winding specifications, running speed and torque, and ambient temperature among others. The motor’s performance documentation will sometimes include this.
Phase shift losses will be determined entirely by the electrical components used in the drive’s construction. The amount of phase shift in any given drive can only be quantified empirically through experimentation. Further details of phase shift effects are well beyond the scope of this article.
EXTENDING BEYOND THE MOTOR
ADDING GEARHEADS TO THE EQUATIONGearheads are commonly attached to motors for many reasons, namely to gain a mechanical advantage or reduce cogging effects. Thankfully, the same power equation that is applicable to motors also applies to gearheads; one just needs to account for the efficiency of the gearhead in addition to all the other components. The power balance equation is extended to the following equation where Pr refers to the gearhead output rather than the motor output:
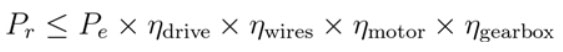
Understanding the previous equations allows a rough calculation of the current drawn from the power supply. In the case of a DC power supply:

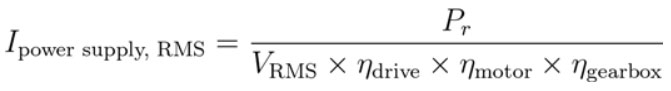
SUMMARY
- Power out of a system can never be more than power into a system.
- Efficiency of each component must be accounted for to find the maximum power a system can output relative to the power brought in.
- This can be a great step to take as a sanity check that you have a properly sized drive for the application since all drives have a power output limit. Typically, this limit is seen in the rated amperage limit.
- Power out of a system can never be more than power into a system.
A lesson for me is that I need to involve you earlier in the program.
You were tireless in your support and it will not be forgotten!
